Euler Home

Search

Geometry with Euler

Euler has some useful functions for geometry. Most functions are implemented in numerical and also in symbolic form. You have to load the geometry file before using the functions.

Geometry in Euler

>load geometry

Numerical and symbolic geometry.

Euler Line

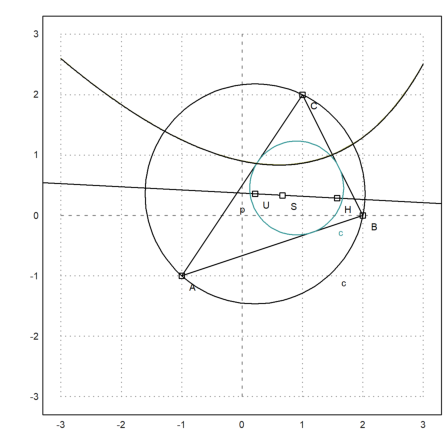
For a demonstration, we compute and plot the Euler line in a triangle. The following construction has been done with C.a.R. and shows the result we wish to achieve.

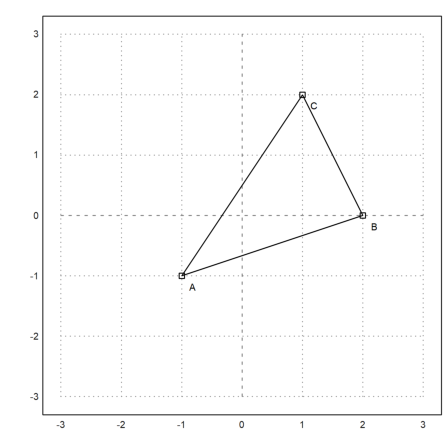
First, we define the corners of the triangle in Euler. We use a definition, which is visible in symbolic expressions.

>A::=[-1,-1]; B::=[2,0]; C::=[1,2];

To plot geometric objects, we setup a plot area, and add the points to it. All plots of geometric objects are added to the current plot.

>setPlotRange(3); plotPoint(A,"A"); plotPoint(B,"B"); plotPoint(C,"C");

We can also add the sides of the triangle.

>plotSegment(A,B,""); plotSegment(B,C,""); plotSegment(C,A,""):

Here is the area of the triangle, using the determinant formula. Of course, we have to take the absolute value of this result.

>&areaTriangle(A,B,C)

                                   7
                                 - -
                                   2

We can compute the coefficients of the side c.

>c &= lineThrough(A,B)

                            [- 1, 3, - 2]

And also get a formula for this line.

>&getLineEquation(c,x,y)

                            3 y - x = - 2

For the Hesse form, we need to specify a point, such that the point is on the positive side of the Hesseform. Inserting the point yields the positive distance to the line.

>&getHesseForm(c,x,y,C), &at(%,[x=C[1],y=C[2]])

                             3 y - x + 2
                             -----------
                              sqrt(10)


                                  7
                               --------
                               sqrt(10)

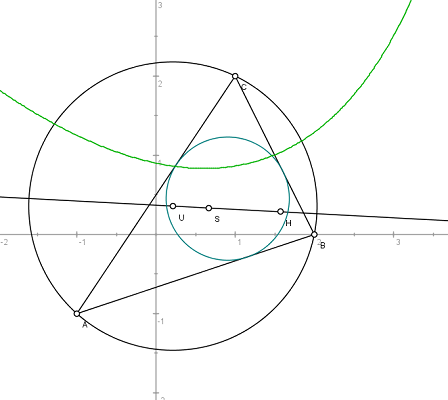
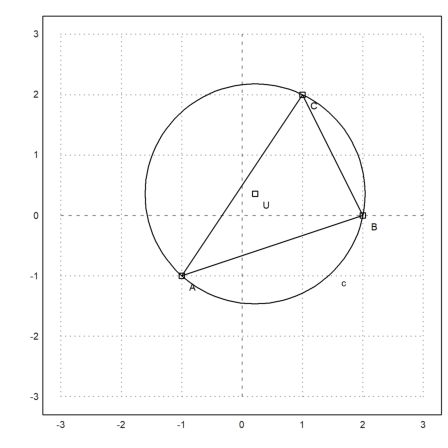
Now we compute the circumcircle of ABC.

>Cu &= circleThrough(A,B,C); &getCircleEquation(Cu,x,y)

                          5  2        3  2   325
                     (y - --)  + (x - --)  = ---
                          14          14     98

>U &= getCircleCenter(Cu)

                                3   5
                               [--, --]
                                14  14

Plot the circle and its center. Cu and U are symbolic. We evaluate these expressions for Euler.

>plotCircle(Cu()); plotPoint(U(),"U"):

We can compute the intersection of the heights in ABC (the orthocenter) numerically with the following command.

>H &= lineIntersection(perpendicular(A,lineThrough(C,B)),...
   perpendicular(B,lineThrough(A,C)))

                                11  2
                               [--, -]
                                7   7

Now we can compute the Euler line of the triangle.

>el &= lineThrough(H,getCircleCenter(Cu)); &getLineEquation(el,x,y)

                            19 y   x      1
                          - ---- - -- = - -
                             14    14     2

Add it to our plot.

>plotPoint(H(),"H"); plotLine(el()):

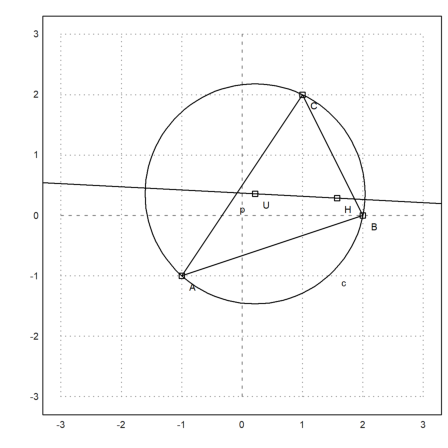
The gravitational center should be on this line.

>S &= (A+B+C)/3; &getLineEquation(el,x,y) with [x=S[1],y=S[2]]

                                1     1
                              - - = - -
                                2     2

>plotPoint(S(),"S"):

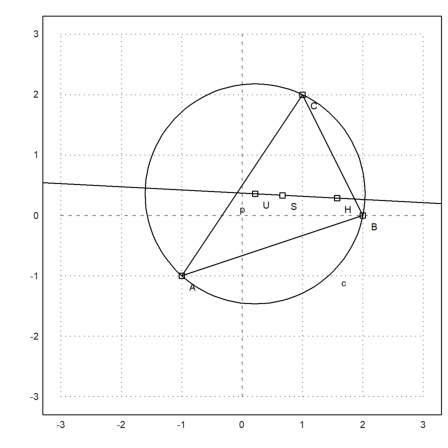
The theory tells us SH=2*SU. We need to simplify with radcan to achieve this.

>&distance(S,H)/distance(S,U)|radcan

                                  2

The functions include functions for angles too.

>&computeAngle(A,C,B), deg(%())

                                    4
                        acos(----------------)
                             sqrt(5) sqrt(13)

60.2551187031

The equation for the center of the incircle is not very nice.

>Is &= lineIntersection(angleBisector(A,C,B),angleBisector(C,B,A))|radcan

           3/2
         (2    + 1) sqrt(5) sqrt(13) - 15 sqrt(2) + 3
        [--------------------------------------------, 
                              14
                                                              3/2
                          (sqrt(2) - 3) sqrt(5) sqrt(13) + 5 2    + 5
                          -------------------------------------------]
                                              14

In Latex, this prints as

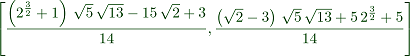
Let us compute also the expression for the radius of the inscribed circle.

>ri &= distance(Is,projectToLine(Is,lineThrough(A,B)))|ratsimp

        sqrt((- 41 sqrt(2) - 31) sqrt(5) sqrt(13) + 115 sqrt(2) + 614)
        --------------------------------------------------------------
                                  7 sqrt(2)

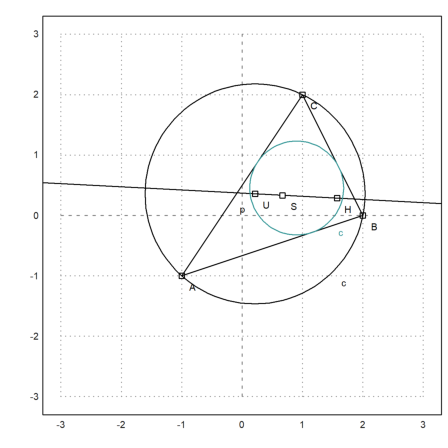
>Ci &=  circleWithCenter(Is,ri);

Let us add this to the plot.

>color(5); plotCircle(Ci()):

A Parabola

Next, we find an equation for the points with equal distance from C to AB.

>eq &= getHesseForm(lineThrough(A,B),x,y,C)-distance([x,y],C)

               3 y - x + 2               2          2
               ----------- - sqrt((2 - y)  + (1 - x) )
                sqrt(10)

Using Euler's level plot, we can add this to plot.

>plot2d(eq,level=0,add=1,contourcolor=6):

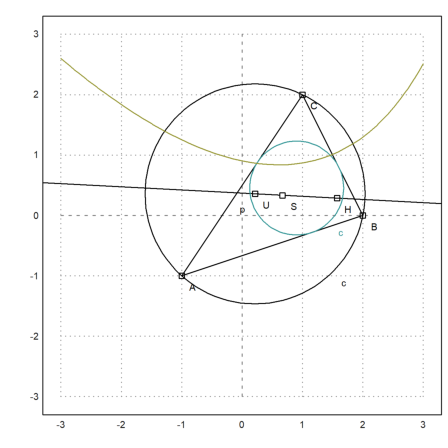
This should be some function, but the default solver of Maxima can find the solution only, if we square the equation. Consequently, we get a fake solution.

>seq &= solve(getHesseForm(lineThrough(A,B),x,y,C)^2-distance([x,y],C)^2,y)

        [y = - 3 x - sqrt(70) sqrt(9 - 2 x) + 26, 
                              y = - 3 x + sqrt(70) sqrt(9 - 2 x) + 26]

The first solution is

Adding the first solution to the plot show, that it is indeed the path we are looking for. The theory tells us that it is a rotated parabola.

>plot2d(&rhs(seq[1]),add=1):

Euler Home